- Vitruvian Man
- Pascal / Shijie Triangle Review
- The Hockey Stick Pattern
- Revealing The Fibonacci Pattern
- Phi - The Golden Ratio
- Binomial Solving
Second Class consists of;
- Workshop Groups
- Binomial Solving - Continued
- Using a System of Equations
First he presented to us 'The Vitruvian Man', which was originally a portrait, drawing, painting... uh.. artistic depiction of man, drawn by none other than Leonardo Da Vinci...
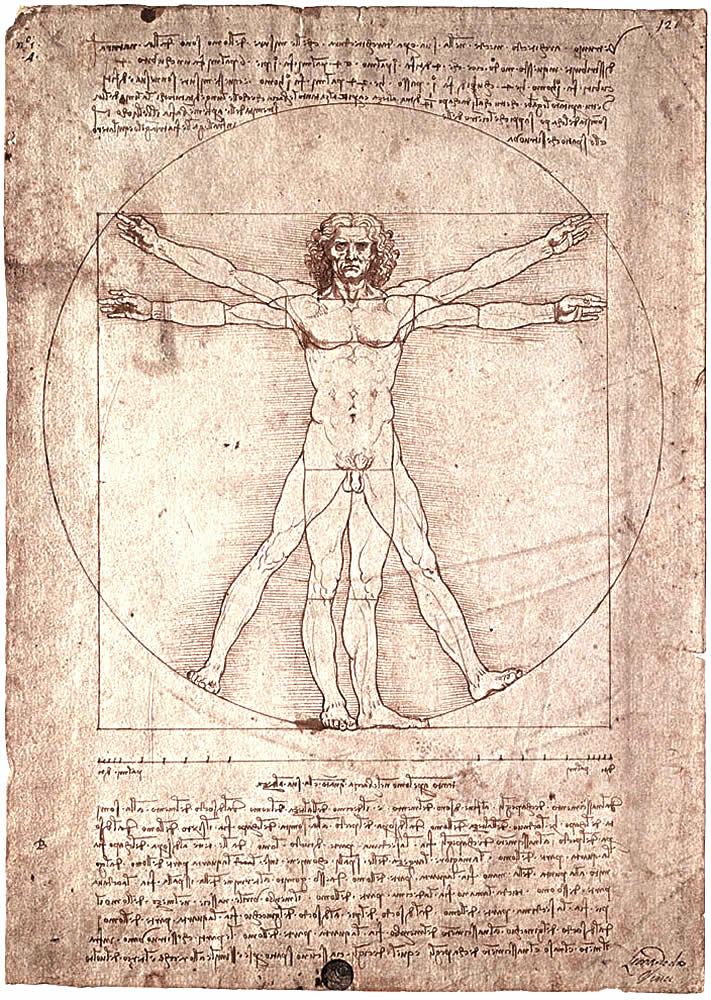
ANYWAYS, The Vitruvian man is the most accurate depiction of man, with everything being of most accurate measurements. Fingers, arm length, leg length etc. I stated that if no mutations had occurred in a human body, A person's Wing Span (Length from arm to the other) is equal to that person's height. And so, Mr. K measured me. Turns out, with my hair, and shoes, I seemed to be longer than my wing span. Subtract my hair and shoes, it would be just about right. He then talked about the symmetry of people and that if someones face or body was more symmetrical, the closer they would be to being "beautiful".
Concluding that, we reviewed 'Pascals' Triangle. Notice the quotations there. We first went over the patterns. Such as...
- when the binomial is expanded, A's exponent is decreasing, and B's exponent is increasing.
- the degree of any term, (a, ab, b) is equal to the term of the binomial.
ex.) (a + b)² = a²+ 2ab + b² <-- the exponent on the A and B terms of AB have a sum of two.
The green line in pascal's triangle are the triangular numbers.
- Justus, [in the previous class] found the tetrahedral numbers. (Follow the link to get a better definition etc)
- The powers of Two are embedded in each row. Example; 2° = 1 (Zeroth Row) 2¹ = 2 (First row) 1 + 1 = 2 ... and so on.
- The powers of Eleven [Word up to Elven] are also embedded in each row.
Now getting on with the Regularly Scheduled programming...
The Hockey Stick Pattern
He showed us new patterns within Zhu Shijie's Triangle. Such as the 'Hockey Stick' Pattern... How Canadian :) Basically, the hockey stick pattern is starting from any side (starting with one), go down diagonally, then cut back in the direction you came in and voila, the sum of those numbers are equal to the last number you cut into. Coincidence? I don't think so.
He showed us that the 'Nacci sequence is found all around us, like in tree's and well, plants. For example. They're found in Pine Cones, Flowers, and their leaves. Bee's also respect the 'Nacci numbers when reproducing, as the male can only have one parent, and the female two, and from there you can pretty much depict how that goes down.
Phi - The Golden Ratio
He then started to divide each Fibonacci number by the number before that one. We found that the resulting number seemed to be reaching a certain number. He then introduced to us... Phi! "PHI is One H of a lot COOLER than Pi!!!" Get it? GET IT?! *crickets* Ok cool
So we have this here. If you want to look into it more, go to http://goldennumber.net/. I believe it's the 'Phinest' Source on Phi, or the Golden Sequence, or Golden Ratio, or ... You get where I'm goin'. So he did some stuff, like explaining that a 4 x 6 picture is better because it approaches Phi more than the 5 x 7 photographs. Apparently if you take pictures like the way he has set up in the slide above (at the black spots), you'll get a better looking, more attractive picture, if your object is concentrated at one of those points. This was where he started to tell us how to get 10 % more on the things we hand in... by making it approach Phi. So then he did all this stuff to 'Build' a golden ratio. So you...- Take a square, cut it in half
- Make a semi-diagonal, in which you get a Pythagorean triangle...
- and then I got lost after that.
He then went to the point where the golden ratio is 1 + √5 / 2 = 0. He then went on to explain Phi is everywhere, in the proportions of your body, (elbow to finger, wingspan, feet, height etc.) Everything is divided Long by Small to get Phi. So basically to get 10% of everything you hand in, hand it in so that it respect's Phi, and it'll look that more attractive... I think.
Basically, the more symmetrical you are, the closer you are to 'beautiful'. This is why a famous building in Athens (My bad, I couldn't recall what the building was called), is crumbling, but still looks appealing to people.
INTERMISSION
He went on an then introduced this...
So then we all screamed in terror (At least I did) as if Mr.K adopted the Dark Side due to this very long expression. But really it's the same thing we've been doing all along. nC2 = N! / (N-2)! 2! = N(N-1)/2! Which we find is one of the expressions within the algebraic expansion. So don't run away.
- The coefficient of the 'ith' term is nC(i-1) ex.) for the 5th term, you'd choose 4
- The exponent on the "a-term" is given by: [n - (i - 1)]
- The exponent on the "b-term" is given by: (i - 1)
- The relation holds for each term in the expansion [exp a] + [exp b] = n
- The number of terms in any binomial expansion is: ( N + 1 )
Remember, There's always one more term than the degree of the binomial.
THE SECOND CLASS
To make this easier, we changed it into the (a + b) format we were using earlier. So we let a = 2 and b = x. We then have it as (a + b) ^7
So to find the 4th term we set it up as so;
t4 = 7C3 a^4 b^3
B's exponent is 3 because of (i - 1), 4 -1 in this case.
A's exponent is 4 because of [n - (i - 1) ], [7 - 3] in this case.
So then we came down with 7! / 4!3! (2)^4(x) ³ <-- We put back in the variables.
Then we came down with 35 (16) (x³) = 560x³
We then did about two more of them, but what I found really cool about how he did one of them is how he multiplied 56 an -8.Basically he broke up 56 into 50 and 6. He then showed the concept of basically doubling those three times (2 cubed).
So first he doubled 50 and 6 --> 50 * 2 = 100 6 * 2 = 12
... then he doubled it again --> 100 * 2 = 200 12 * 2 = 24
... then he doubled it again --> 200 * 2 = 400 24 * 2 = 48
then he added them together --> 400 + 48 = 448.
And because the 8 was negative... --> -448.
So try it on your calculators... 56 * -8 = ... - 448!
Yeah I know, crazy.
Ok, lets try to wrap this up quick. I'm just showing this slide, as it's many student's mistakes to multiply the -1 by 3, but really, you're just cubing it... which still makes it -1 :)
Here, we discussed finding a certain term within an expansion.
First we did it a long and tedious way ( I didn't put up the slide for it), and once we found the pattern, which is the exponent changing by the same difference, we can then find the term we are looking for. But Mr. K showed us a more elegant way.
First, we wrote out the way we'd choose a number from an amount. And we'd use dummy variables so that we can just get the structure up.
So we set it up like ; qCp a^p b^q
This is where our *Remember* segment comes in.
REMEMBER, the exponent's sum must match the degree of the Binomial! So take a quick look above in the slide and we notice the exponent of the Binomial is 9, therefore, p + q MUST, and I mean MUST equal 9. Now let's put back in the variables so we can solve this little sucker.
(x^3) ^p (-x^-1) ^q So since we put back in the variables, the exponents now are 3p and -q. So then because we're looking for x^7, we set it up like;
3p - q = 7. well at least it should equal 7, because that's how we built it.
Then create a system of equations. p+q + 3p - q = 9+7 --> 4p = 16. Therefore, p = 4 and since p = 4, and the exponents MUST equal 9...
9 - 4 = 5. Therefore q = 5.
Now we can find that term.
9C5 (x^3)(-x^-1)^5 = x^7
= 126x^7
Class, this concludes today's lesson and work.
REMEMBER!
The sum of the exponents on any term MUST equal the degree of the Binomial!
Don't mistake exponents and multiply the coefficient. It must be raised to that exponent.
Don't forget about the Zeroth term!
And finally... A LOGARITHM IS AN EXPONENT! ... Just in case you forgot.
A side note;
Watch Iron Man. It's Dope. ... or should I say Phi? Oh yeah before I forget. The Next Scribe is ZEPH.
Now then, I gotta get some sleep and stay PHI! 8)
Rence ~ Out
No comments:
Post a Comment