Pascal's Triangle That Wasn't Really Pascal's Triangle...

Hi everybody...Benofschool here to scribe what we did today in class. Today Mr. K promised us that today's class will show a way that G-D built the universe and that it will blow our minds. But we didn't have enough time so we didn't get to G-D building the universe but the lesson did blow our minds.

Lets start with the first slide. The first slide had a picture of an ancient Chinese diagram. Hey doesn't it look a bit like Pascal's Triangle? Well it was Zhu Shijie's triangle created 400 years before Pascal.
When I asked my grandfather what the characters meant but unfortunately he said he couldn't understand it. The characters were of the ancient Chinese characters. The Chinese language has evolved a lot. But my grandpa and I found a connection of why the number 7 (fourth character from the left) was there. It probably had to do with how many rows the triangle had but there seems to be something different about the first 4 rows because the last row on the triangle says something about the number 7.

After a little conversation about that triangle and teaching Mr.K some Chinese pronunciation lessons we continued onto the next slide. He asked us to expand and simplify the binomials. It was a bit tedious but we did it. Since some of them were a bit long he enlarged the hidden answers like he always does (that sneaky guy...). It looks like any other expansions we've done but there was something special about them. So let's look at the next slide. It may give us some insight into what was so special about those binomials.

On the next slide...
we were asked to find the next two rows from what was shown. It was pretty easy to see. To find the next row just add the numbers on the previous row to obtain the next row for example: since there are no numbers before the 1st 1 and after the last 1 we can assume there is a zero there so 0 + 1= 1 so the next row starts with a 1. The next number can be obtained by adding 1 and 4 to get 5 on the next row. 4 + 6 to get 10 to get the next number, and so on until we get to
1+ 0=1 that ends the row.

So if we look at the numbers in each row and compare them to the coefficients of the simplified binomials on the last slide, they are the same. Lets look at (a+b)³: we get that the coefficients are 1,3,3,1 just like on the 4th row of the triangle. But wait that's not all of the patterns found on the binomial work. If we look at the exponents of the variables a and b. The coefficients of a decreases counting from the exponent on the original binomial until the exponents is zero. The coefficients of b are increasing until we get to the exponent on the original binomial. We were all commenting on how much more easier that would be to expand those binomials if we knew that patter so we went on to finding (a+b)^6.

Then on the 4th slide...

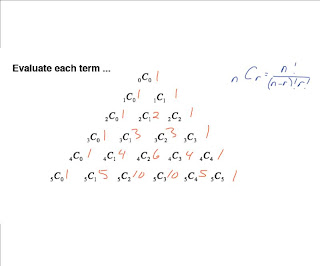we were asked to evaluate the following. So to do that all we need to do is to plug it into our
TI-Calcumulators or just plug it into the Choose or Combination formula seen on the picture to evaluate it and it seems the numbers were the same on the triangle. Cool huh? Pascal originally "made" the triangle to help a friend gamble. Gambling is bad...

The next few slides we were just looking for more patterns on "Pascals" Triangle. We found the counting numbers (in yellow). On the other slide we found the powers of 2 on the triangle. If we find the sum of the numbers on each row we get a power of two. Cool. And the digits of the power of 11 is found on it too but a few rows down there is a problem... or is there? Since the 10 is on the 6th row we carry that one to the 10 on the left which makes it 11 and then we carry that 1 to the 5 to the left and we get six so 11^5 is 161051. Those slides totally blew our minds...

Okay for some Pascallian/ Zhu Shijie vocabulary...
Rows- The row of numbers: First Row, Second Row
Terms- The terms are the numbers on each row: First Term, Second Term
That vocabulary only works in high school. In University the first row is referred to as the zeroth row and the first term is referred to as the zeroth term. So if we combine the high school vocabulary the 5th term on the 3rd row is the number 6.

A little challenge for tonight is to find the Fibonacci Sequence (1,1,2,3,5,8...) on the triangle. And homework I thought will be posted on the blog but there doesn't seem to be any homework. I guess homework should be exercise the next like Mr.K always says and get practice on the unit because the pretest is on Wednesday and the test is on Thursday. So get those BOB's posted. Monday will be the day that Mr.K will show a way that G-D built the universe.

I just proved to my parents that the male seahorses give birth. The next scribe will be Rence. Until next class good bye and good night....